For convenience of reasoning we left out the spring until now. Without a spring however the parallel plate capacitor has no use. Every charge on the plate results in an attractive force, which will move the plates to each other until they touch. So you need another element to reach a state of equilibrium. You can realize that by means of a mechanical spring, as is drawn in Figure 2.1.1(a). The figure is repeated in Figure 2.5.1.
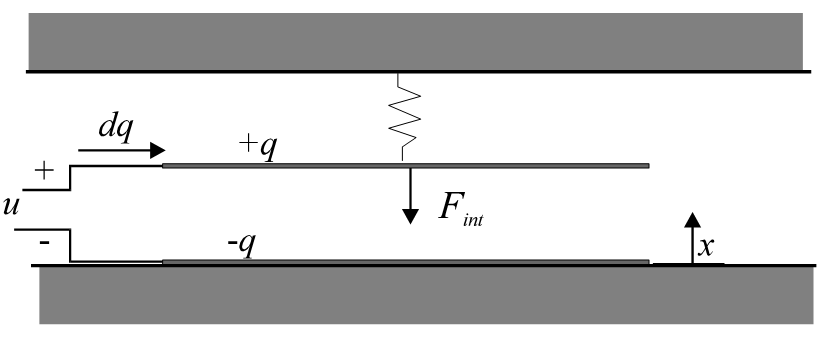
Figure 2.5.1: Capacitor with one electrode suspended by a spring.
We now have to include the force of the spring when calculating the integral (2.16) to find the energy stored in the transducer. If x0 is the equilibrium position of the moveable capacitor plate, the external force needed because of the spring is given by:
|
(2.22) |
where K is the spring constant.
To find the energy stored in the transducer we start with equation (2.15) which gives the change in energy due to work done at the ports:
|
(2.23) |
As before, we integrate (2.23) along a path, from the point where both x=0 and q=0 up to the point (x, q) where we want to know the energy:
|
(2.24) |
We first integrate over x, while keeping q equal to zero. Next, we integrate over q, keeping x constant. We could also do this the other way around, but this is more complicated because doing the integral over x with charge on the capacitor means that we need an expression for the electrostatic force.
Performing the integration we find the following expression for the energy, ignoring any constant terms as these do not have any influence on the partial derivatives:
|
(2.25) |
where the first term represents the energy stored in the electric field and the second term represents the energy stored in the spring.
We now find the force and the voltage by differentiation, just as before,
|
(2.26) |
|
(2.27) |
You have to interpret (2.26) as follows: When you have a capacitor and a spring of the given geometry and you bring a charge q on the capacitor, then you will need to apply the force Fext to keep the plate distance equal to x. Or to put it in a different way: with this x and q the system exerts an internal force on the capacitor plate given by Fint = -Fext. You need the external force Fext to prevent the plate from moving. There is an equilibrium situation where an external force is not needed (Fext = Fint = 0). We can calculate the equilibrium position by equating (2.26) to zero and solving for x. The result is:
|
(2.28) |
Note that in this case we can easily calculate the equilibrium position because the electrostatic force is proportional to q2, but independent of x. So we can easily find the position xeq where the spring force is equal but opposite to the electrostatic force. This will be different if the capacitor is controlled by a constant voltage instead of a constant charge as we will see in the next section.
You can also look at the system in another way. Equation (2.25) gives the total potential energy for a system consisting of two components, a capacitor and a spring. From this we have calculated the force and the voltage as a function of the variables x and q. We can also say that the capacitor is the transducer and that we have coupled it to a spring, i.e. we can treat the spring as if it is outside the transducer. In that case the spring will deliver (part of) the external force (2.19) that we calculated for the capacitor without spring. Equating the force of the spring (2.22) to the external force given by (2.19) will give exactly the same equilibrium position (2.28). Unraveling a system into its basic components has considerable advantages when the system becomes complicated. To do this a number of methods have been invented. One of them, the bond graph method, is very powerful because it works in all physical domains.